Usage Notes
Mathieu's equation is
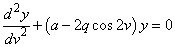
It arises from the solution, by separation of variables, of Laplace's equation in elliptical coordinates, where a is the separation constant and q is related to the ellipticity of the coordinate system. If we let t = cos v, then Mathieu's equation can be written as
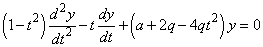
For various physically important problems, the solution y(t) must be periodic. There exist, for particular values of a, periodic solutions to Mathieu's equation of period kπ for any integer k. These particular values of a are called eigenvalues or characteristic values. They are computed using the routine MATEE.
There exist sequences of both even and odd periodic solutions to Mathieu's equation. The even solutions are computed by MATCE. The odd solutions are computed by MATSE.
|
PHONE: 713.784.3131 FAX:713.781.9260 |